 |
 |
 |
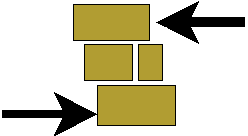
A stone work structure with no shearing forces is safe. The more shearings there are, the more likely the structure will fall.
 |
 |
Viollet le Duc thought that shape (i.e. structure) should be adapted to the materials used. He appraciated gothic architecture as the most effective technic ever found to build stone cathedrals (large, high and lighty building). Gaudi founded a way even more effective for stone constructions. His way can be considered as the most effective way using some physical properties (it minimizes the shearing forces which are the causes of collapses). He found the way not using math as I will to demonstrate what I do assess but models that took naturally the optimum shape by putting them upside down. As you know it, Gaudi was very fond of nature and nature inspires his work very much. Once again natural shapes have proven to be effective and tightly linked to efficient structures.
Gaudi used models in several buildings including the Colegio Teresiano, The Guell crypt, the Sagrada Familia (only towers have been built so the "parabolic" structure is not visible). The "parabolic" archs are used in almost every building by Gaudi.
 |
 |
 |
A stone work structure with no shearing forces is safe. The more shearings there are, the more likely the structure will fall.
 |
 |
Gaudi used some models to test his structures. I have seen few pictures of them but I
have never read any description of there fabrication. I have some ideas about it and I
will try to explain how Gaudi or anyone else could have "calculate" a safe large
stone structure without any computer.
Take chains with little link, glue, a large board. That's all. Oh, I was forgotten that
you need not to be on the space shuttle. Just stay on earth, it will be easier than on the
moon.
The trick to change compression into extension is to use gravity force. It is to put the
stone work structure upside down to obtain a chain structure and reciprocally. 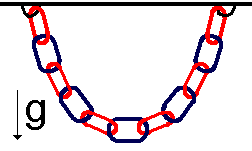

It is the first exercise with our model, making the simplest stone structure: an arch. Take a chain with small links and glue its two extremities to your bedroom ceiling (it works also in your bathroom). You will find thanks to our transformation above (i.e. downside up) an optimal shape for your arch. Playing with the length of the chain, and the distance between the two extremities, you can obtain different shapes for arches.
The system is constitued by the ceiling (an horizontal plan named P), Two glue points G and G' that belong to P, and a chain with "very little links" that is glued by its two extremities to G and G'. is the curve absice of the chain, is the linear mass. The system has two orthogonal symetry plans which are (the set of points at equidistance of the two glue points G and G') and P
2 (the spawn(ed?) by and , and at home it's the plan of your computer screen). Of these considerations of symetry, We can conclude that the solution is also include in the plan P2 (i.e. your screen) and is symetrical by the mediatrice of [GG'] (i.e. the vertical line going by the middle of GG'). Two axes: horizontal ox, vertical oy with their associated unity vectors andLet us consider the subsystem of the chain between -l and l. This system is also symetrical and immobile. We can apply to it the law of the equilibrium of forces. gravity P=-2lmlgj, F(l)=Fx(l)i+Fy(l)j and F(-l)=Fx(-l)i+Fy(-l)j. Projecting on the axe oy, we get the equation: -Fy(-l)+Fy(l)-2lmg=0. We can add for symetry reasons that Fy(l)=-Fy(-l) so the equation becomes 2Fy(l)=2lmg that is Fy(l)=lmlg.

Let us now consider the sub system composed of only one link. It is still in equilibrium so on ox axe we get Fx(l)-Fx(l+dl)=0 i.e. Fx(l)=Fx(l+dl) from link to link, we have Fx(l)=C1 whatever the value of l.
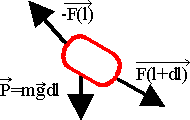
As the link is small, the tangente at point P(l) (called T(l)) is colinear to F(l). So we can write the definition of colinearity between T(l) and F(l):
(1) y'(x)=dy/dx=C2*mlgl/C1
We now aim at eliminating l in function of y and x. We will use the Pytagorus theorem dl2=dx2+dy2. This leads to dl/dx=
sqrt(dy/dx2+1) . We can derive (1): y''(x)=C2/C1*mgdl/dx and use the expression of dl/dx to give(2): y''(x)=C3*
sqrt(dy/dx2+1) We will now make a variable change: we can assume that a function t exists where y'(x)=sh(t(x)) (sh is the hyperbolic sinus). That implies y''(x)=t'(x)ch(t(x)) applying the drivation law for conpouded functions. (2) can be rewrote as
(3) t'(x)ch(t(x))=C3*
sqrt(sh2(t(x))+1) Now let us use the relationship between sh and ch: ch2-sh2 =1. we get
(4) t'(x)=C3
so t(x)=C3*x+C4 and replacing t in (3)
(5) y'(x)=sh(C3*x+C4)
Finally y(x)=C5*ch(C3*x+C4)/C3. And because y(0)=0 we can calculate that C4=0. So
y(x)=K*ch(C3*x) where K=C5/C3.
Notes and Definitions:
The former calculus took into account only the structure. It is possible to take into account also the filling walls. It is to change the link mass from ml g dl into ml g dl+mwy where mw is the mass per surface unit of the wall. This modifies (1) into
(1') y'(x)=dy/dx=C2*mlgl/C1+C2*mwy/C1
I do not know how to solve simply those kind of differential equation but Gaudi knew how to do it without math: he evaluated the mass of the wall and then hang some little masses to the links to simulate the weight of the walls.
I hope you had fun in reading this.
 |
The Art-Nouveau-round-the-world server is dedicated to promote a better knowledge of the Art Nouveau period (1890-1914) |